Music and Crop Circles
The same principles which govern the intervals of musical notes are often observed in the organization of crop circles, leading many to believe that the crop circles have musical meanings. There are countless methods of associating the geometry of crop circle designs with those of musical scales. Some of the more popular associations are listed below:
 |
A simple design such as this will also produce a simple musical chord. The outer circle is twice the diameter of the middle one, and the middle one is exactly in between. This means that they are at the ratios of 1, 3/2, and 2, which will produce a 1, 5, and octave musical chord. |
 |
This other simple design (one of the first designs studied by Delgado), also represents a simple harmonic ratio. The first orbiting sphere is located at 90 degrees, while the second is at 180 degrees, giving a 2:1 or octave ratio. This method of organization can quickly produce more interesting patterns as orbital elements are spun around the parent. |
 |
Once again, a simple design yields music. If the intervals of the diatonic scale are used to mark spots around the edge of a circle, you will find that there will be markings at 45, 90, 120, 180, 240, and 315 degrees. This is triangle has its corners at 120, 240, and 360 degrees, which, when equated to music, would produce a C-F-A chord (assuming key is C major). |
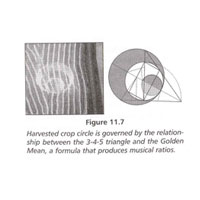 |
This final design is taken from Freddy Silva's book, and it illustrates the intense geometric values behind an actual crop circle. The ratios present (3:4:5) offer many musical interpretions. The numbers could be rearranged into 4:3, 5:4, and 5:3 which would give a third, fourth, and sixth (E, F, A). |
There are many more methods of associating the visual patterns of crop circles with the aural patterns in music, but in my research I did not discover many theories that related to the modern, equal tempermant scale of music. A technique used by early 20th century composers called set theory is one that I think yields vast potential for analysis in design The two diagrams below show the method of note arrangement in set theory, and its obvious design implication.
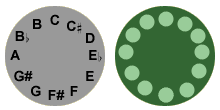
Listen to the audio created by these designs and others
